f(x+) and f(x-) exists at every point x of (a,b) and sup f(t) =f(x-)is less than equal to f(x)is less than equal to f(x+)=inf f(t) | Limit and Continuity | Real Analysis

Description

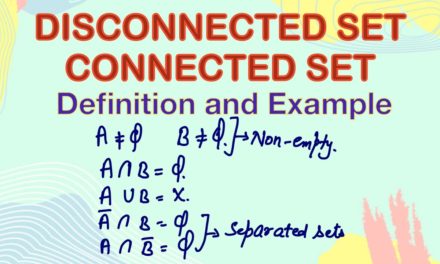
f is monotonically increasing function defined on (a,b) then f(x+) and f(x-) exists at every point x of (a,b) and sup f(t) =f(x-)is less than equal to f(x)is less than equal to f(x+)=inf f(t)
f is monotonically increasing function defined on (a,b) then f(x+) and f(x-) exists at every point x of (a,b) and sup f(t) =f(x-)is less than equal to f(x)is less than equal to f(x+)=inf f(t) pdf
f is monotonically increasing function defined on (a,b) then f(x+) and f(x-) exists at every point x of (a,b) and sup f(t) =f(x-)is less than equal to f(x)is less than equal to f(x+)=inf f(t) handwritten notes

PDF Notes