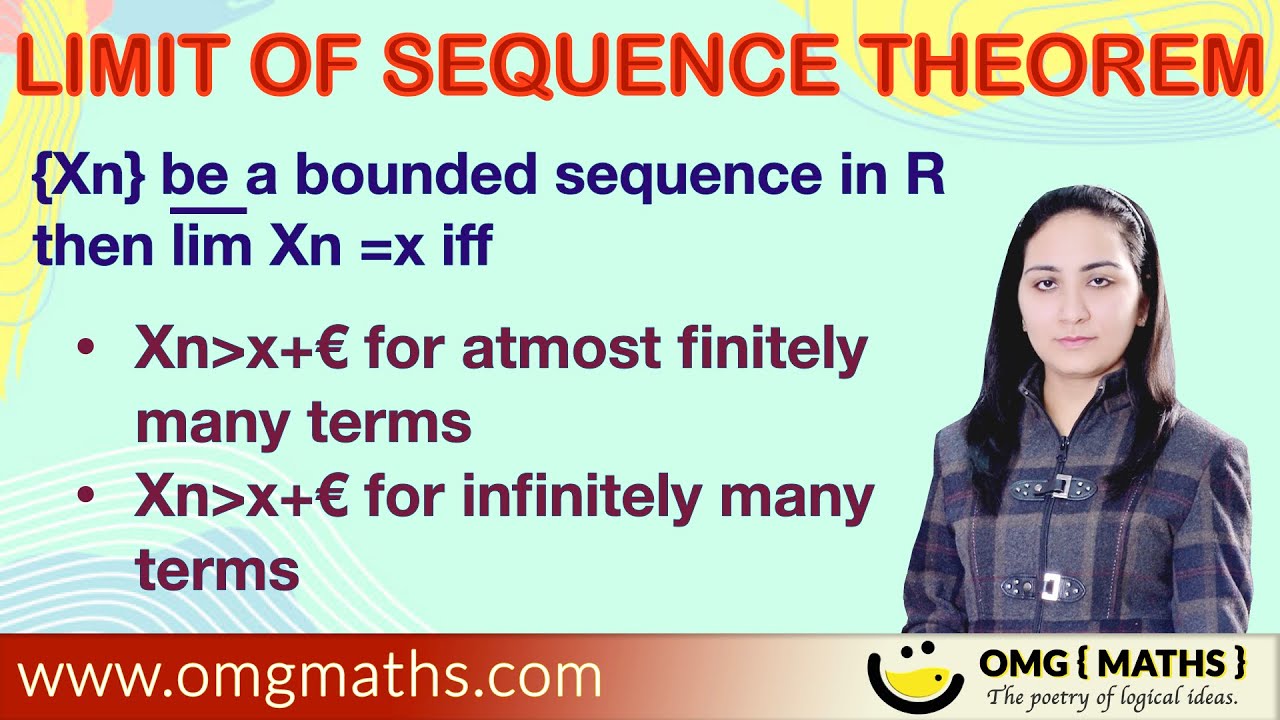
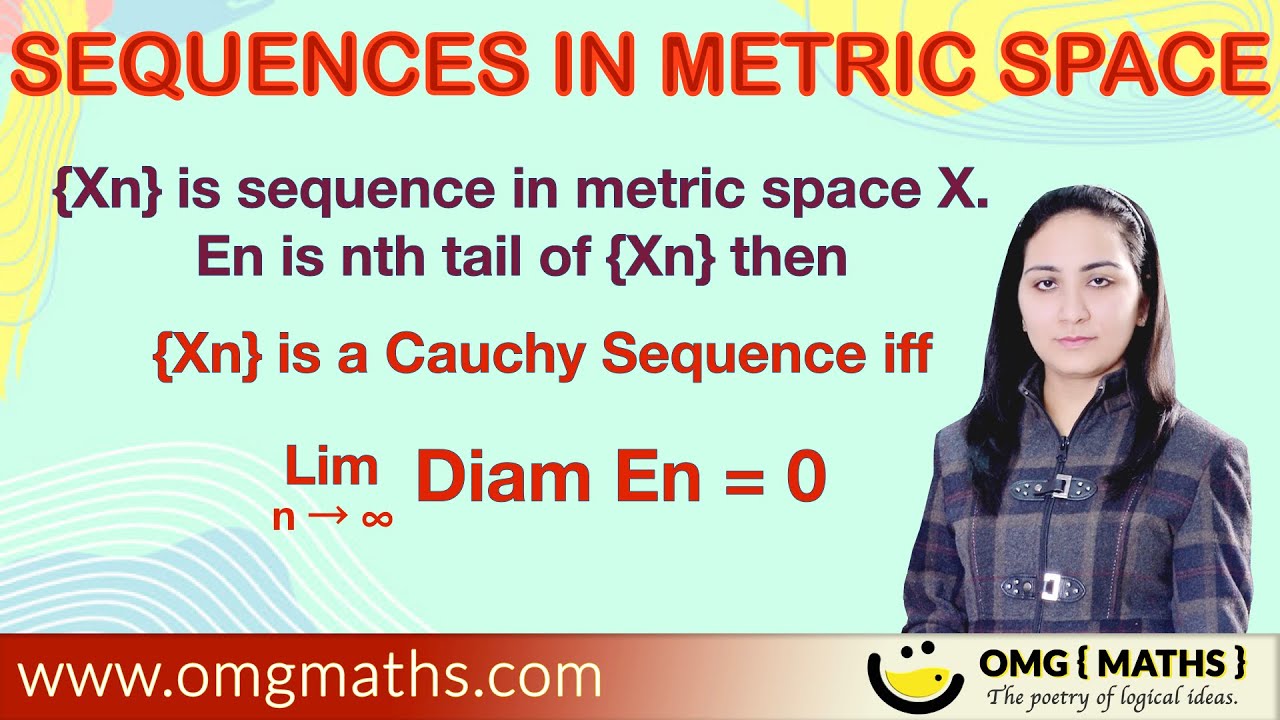
Limit Inferior and limit Superior theorem Pdf Xn is a bounded sequence in R limit superior of Xn is x iff Xn>x+€...
Xn is a bounded sequence in R limit superior of Xn is x iff Xn>x+€ for atmost finitely many terms Xn
read more